Statistically Solving Nonlinear Problems
Recovery in Optical Imaging
Inverse problems in optical imaging seek to recover an object from measurements. Typically, it is done by minimizing a data-fiiting cost between a forwad model of the sought variables and the acquired measurements. However, measurments are stochastic. This randomness is sometimes modeled by randomness in the imaging system or sensor noise. To address measurment uncertinty, a statistical solution can be used. This statistical approach becomes even more significant where the inverse problem is nonlinear.
Stochastic Recovery of Complex-Valued Objects
Light is a complex-valued field. The intensity and phase of the field are affected by imaged objects. However, imaging sensors measure only real-valued non-negative intensities. This results in a nonlinear relation between the measurements and the unknown imaged objects. Moreover, the sensor readouts are corrupted by signal-dependent Poissonian-distributed photon noise. In our paper "Towards A Most Probable Recovery in Optical Imaging", we generalize annealed Langevin dynamics to stochastically solve fundamental nonlinear problems of optical imaging, including phase retrieval, Fourier ptychography, and Poisson denoising. This work was led by Nadav Torem and in collaboration with Prof. Yoav Schechner and Prof. Michael Elad.
Plankton in-situ Tomography: Motivation and Context
Plankton are important to study for their role in both local and global ecosystems. These organisms are vital to the ecology of the planet, as they play a critical role in producing breathable oxygen (of which 50% is due to marine phytoplankton) and serve as the base of the marine food web. To study them outdoors, these translucent specimens are imaged in-situ, where each specimen appears only once before drifting away. In our paper "Plankton Reconstruction through Robust Statistical Optical Tomography", we developed a novel CT modality for plankton 3D tomography using such a random ensemble to statistically estimate an average volumetric distribution of the plankton type and specimen size. It was done in collaboration with Prof. Jules Jaffe and Dr. Eric Orenstein from Scripps Institute of Oceanography, San Diego, California.
Publications
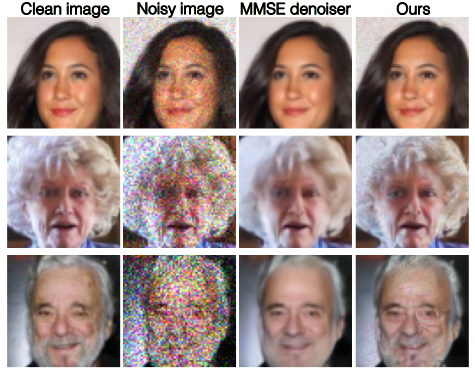
Nadav Torem*, Roi Ronen*, Yoav Y. Schechner and Michael Elad, "Towards A Most Probable Recovery in Optical Imaging", ICCV Workshops, 2023.

Roi Ronen, Yacov Attias, Yoav Schechner, Jules Jaffe and Eric Orenstein, "Plankton Reconstruction through Robust Statistical Optical Tomography", JOSA A 2021, Emerging Researcher Best Paper Award (Oral ICCP'21).